Euclid: impact of nonlinear prescriptions on cosmological parameter estimation from weak lensing cosmic shear
| Authors: |
Martinelli, M.;Martins, C. J. A. P.;Nesseris, S.;Sapone, D.;Tutusaus, I.;Avgoustidis, A.;Camera, S.;Carbone, C.;Casas, S.;Ilić, S.;Sakr, Z.;Yankelevich, V.;et al. |
| Journal: |
|
| Year: | 07/2020 |
| Download: | Inspire| Arxiv |
Abstract
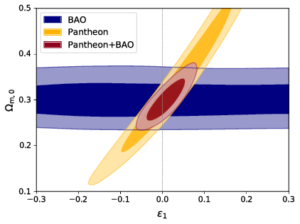
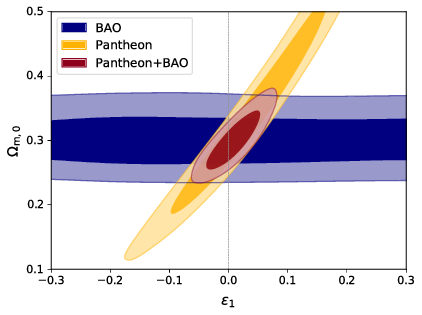
In metric theories of gravity with photon number conservation, the luminosity and angular diameter distances are related via the Etherington relation, also known as the distance-duality relation (DDR). A violation of this relation would rule out the standard cosmological paradigm and point at the presence of new physics. We quantify the ability of Euclid, in combination with contemporary surveys, to improve the current constraints on deviations from the DDR in the redshift range 0<z<1.6. We start by an analysis of the latest available data, improving previously reported constraints by a factor of 2.5. We then present a detailed analysis of simulated Euclid and external data products, using both standard parametric methods (relying on phenomenological descriptions of possible DDR violations) and a machine learning reconstruction using Genetic Algorithms. We find that for parametric methods Euclid can (in combination with external probes) improve current constraints by approximately a factor of six, while for non-parametric methods Euclid can improve current constraints by a factor of three. Our results highlight the importance of surveys like Euclid in accurately testing the pillars of the current cosmological paradigm and constraining physics beyond the standard cosmological model.