The Point Spread Function (PSF) is the response of an optical instrument to an impulse. It characterizes how much the observed light distribution has been distorted by the instrument. Therefore knowing the PSF is fundamental in astronomy for morphometric and photometric measurements.
Multiple frame PSFs super-resolution
Unresolved object like isolated stars give a measurement of the PSF. However, raw telescope images might be undersampled, in which case stars images can not be directly used for galaxies images processing or analysis. The software SPRITE precisely enables one to compute a well-sampled PSF from noisy and undersampled measurements of the same PSF.
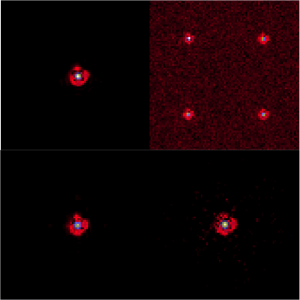
An example is shown here: the top left panel shows a simulated Euclid telescope optical PSF and the top right panel shows 4 randomly shifted low resolution (LR) and noisy versions of this PSF; the bottom left panel shows the PSF estimated from these noisy LR images using SPRITE and bottom right panel shows the PSF estimated using the software PSFEx.
Single frame PSFs field joint super-resolution
The SPRITE method assumes that several measurements of the same PSFs are available. This is never the case in practice because the PSF varies across the telescope field-of-view (fov). The need for several LR images of the same PSF for enhancing the resolution can be relieved thanks to the PSFs field regularity, if one jointly estimates all the PSFs. This is the basic principle of the Resolved Components Analysis (RCA).
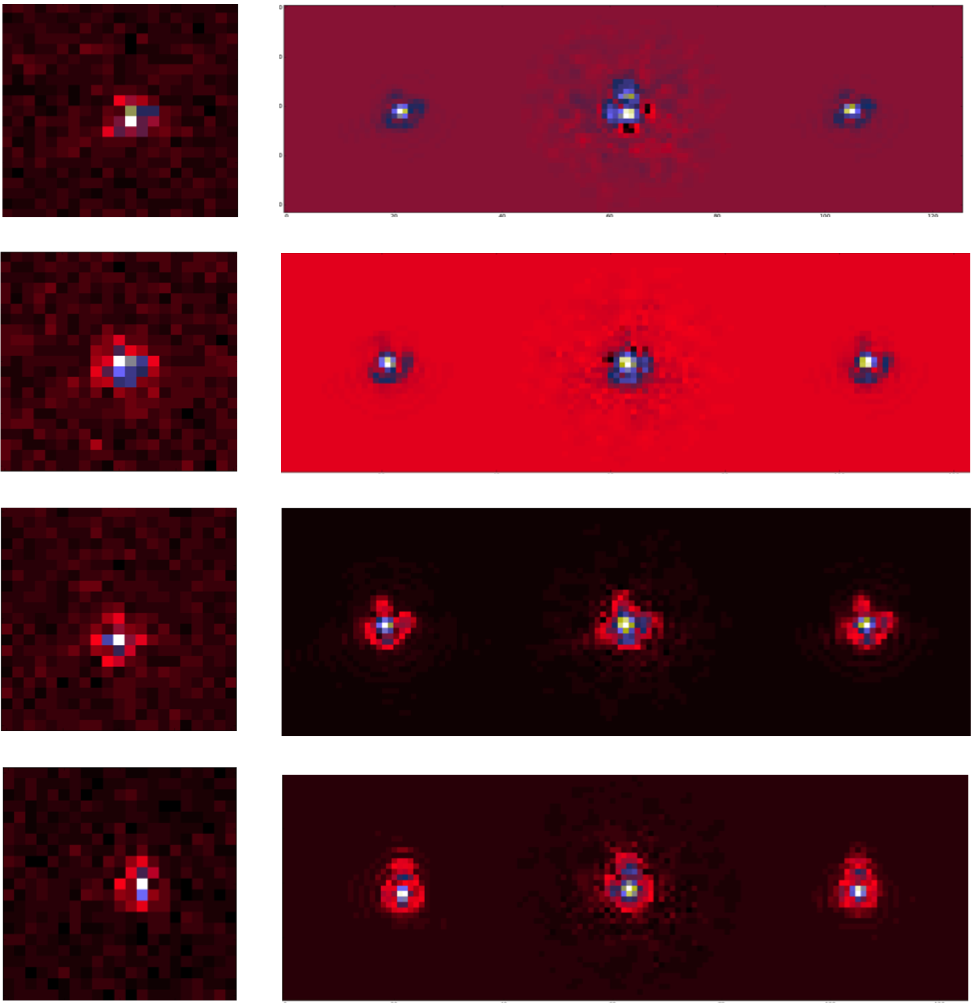
Monochromatic Euclid PSFs restoration. From the left to the right: observed PSFs, original PSFs, PSFEx restored PSFs, RCA restored PSFs.
PSFs field spatial interpolation
SPRITE and RCA provides an estimate of the PSFs at observed stars locations. However, one ultimately needs the PSFs at galaxies locations, which thereby have to be interpolated from the observations related one. In the case of the Euclid telescope, the PSFs morphology significantly changes across the fov. Therefore, it is suitable to use a mass preserving interpolation framework that adapts to non-linear warping. This makes the Optimal Transport (OT) theory, and especially the concept of Displacement Interpolation (demonstrated in the video bellow) particularly appealing. A more complete PSFs interpolation methodology using OT as well as the codes will be made available shortly.
Displacement interpolation between two Euclid PSFs.